Speaker: Felipe-Reyes Osorio, University of Delaware
Image
When
11 a.m. – Noon, Oct. 3, 2024
Where
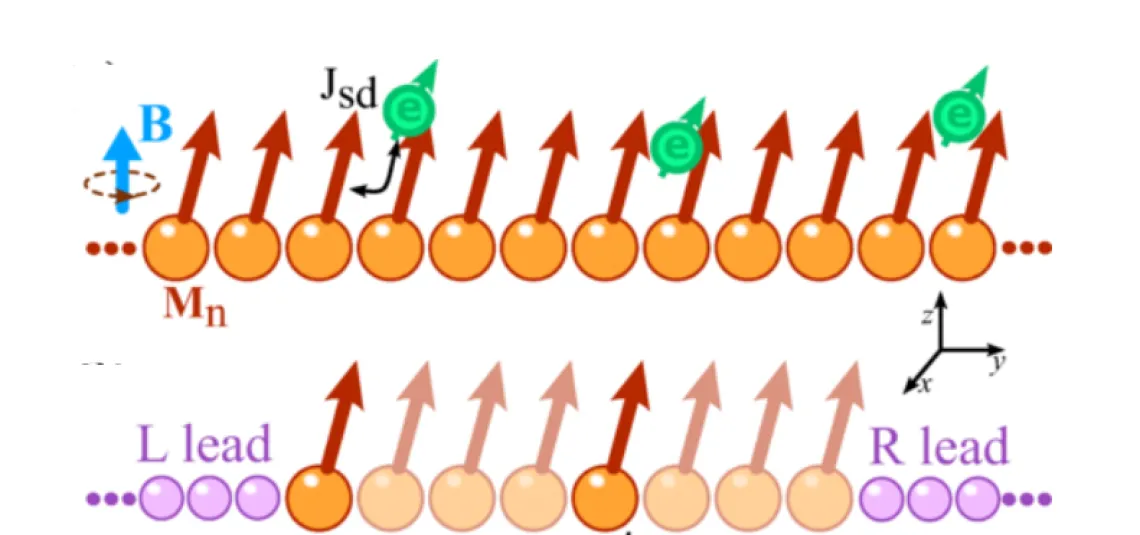
TITLE: Schwinger-Keldysh field theory of nonlocal magnetic damping and its effect on spin waves and skyrmions
ABSTRACT: The nearly ninety-year-old phenomenological Landau-Lifshitz-Gilbert (LLG) equation is widely used in micromagnetic and atomistic spin dynamics simulations to model a myriad of experiments and devices in spintronics, magnonics, and magnetic transport. Despite being a classical framework rigorously justified only for large spins or for ferromagnetic systems, the LLG theory remains a first-line approach due to efficient numerical integration schemes. However, many novel experiments require extending the LLG equation according to the specific microscopic description of the problem. For example, recent experiments on magnetic-insulator/metal bilayers hosting spin waves with dramatically enhanced damping that is also wavevector-dependent add to existing evidence for electronically-induced nonlocal damping. In this talk, we present an extended LLG equation containing nonlocal damping obtained from Schwinger-Keldysh field theory by rigorously integrating out the quantum itinerant electrons within an sd model. Nonlocal damping is operative even if the absence of noncollinearity and spin-orbit coupling. Our extended LLG equation is benchmarked against numerically exact quantum-classical simulations, and compared to prior theories of nonlocal damping. Finally, the wavevector-dependent enhancement of spin wave damping is shown to be compatible with the recent experiments, and a new type of skyrmion mass due to surrounding electrons is also predicted
PAS 236 @ 11:00 AM - 12:00 PM
In-person only, not available via Zoom.
Contacts
Weigang Wang



